Question

If x varies jointly as y and z,and x=8 when y=4 and a=9,find z when x=16 and y=6​

Answer 1

z=12

Explanation:

It is given that x varies jointly as y and z, it means

`x\propto yz`

`x=kyz`

`x(y,z)=kyz`

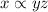
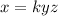
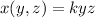
where, k is the constant of proportionality.

It is given that x=8 when y=4 and z=9

`(8)=k(4)(9)`

`8=36k`

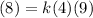
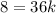
Divide both sides by 36.

`(8)/(36)=k`

`(2)/(9)=k`

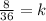
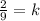
The value of k is 2/9.

`x=(2)/(9)yz`

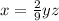
We need to find the value of z when x=16 and y=6​.

Substitute x=16 and y=6 in the above equation.

`16=(2)/(9)(6)z`

`16=(4)/(3)z`

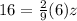
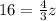
Multiply both sides by 3.

`48=4z`

Divide both sides by 4.

`12=z`

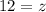
Therefore, the value of z is 12.