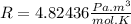Question

If you are given an ideal gas with pressure (p)259,392.00 pa and temperature (T)=200°c of 1 mole Argon gas in a volume 8.8dm3,calculate R to the correct number of significant figure and units under given conditions

Answer 1

Answer:
`R=4.82436 (Pa. m^(3))/(mol. K)`

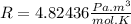
Step-by-step explanation:

The Ideal Gas equation is:

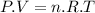`P.V=n.R.T` (1)

Where:

`P` is the pressure of the gas

`n` the number of moles of gas

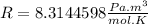`R=8.3144598 (Pa. m^(3))/(mol. K)` is the gas constant

`T` is the absolute temperature of the gas in Kelvin.

`V` is the volume

It is important to note that the behavior of a real gas is far from that of an ideal gas, taking into account that an ideal gas is a single hypothetical gas. However, under specific conditions of standard temperature and pressure (T=0\°C=273.15 K and P=1 atm=101,3 kPa) one mole of real gas (especially in noble gases such as Argon) will behave like an ideal gas and the constant R will be
`8.3144598 (Pa. m^(3))/(mol. K)`.

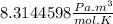However, in this case we are not working with standard temperature and pressure, therefore, even if we are working with Argon, the value of R will be far from the constant of the ideal gases.

Having this clarified, let's isolate
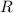`R` from (1):

`R=(PV)/(nT)` (2)

Where:

`P=259392 Pa`

`n=1 mole`

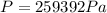
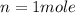
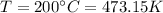`T=200\°C=473.15 K` is the absolute temperature of the gas in Kelvin.

`V=8.8 dm^(3)=0.0088 m^(3)`

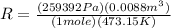`R=((259392 Pa)(0.0088 m^(3)))/((1 mole)(473.15 K))` (3)

Finally:

`R=4.82436 (Pa. m^(3))/(mol. K)`