Question

3) in clinical trials of Nasonex, 3774 adult and adolescent allergy patients (ages 12 and older) were randomly

divided into two groups. The patients in Group 1 (control group) received the placebo, while the patients
in Group 2 (experimental group) received 200 ug of Nasonex. Of the 1671 patients in the control group.
368 reported headaches as a side effect. Of the 2103 patients in the experimental group, 494 reported
a side effect. At the 10% significance level, is there sufficient evidence to conclude that the
nicers that experienced headaches as a side effect is greater than the proportion of
proportion of Nasonex users that experienced headaches as a side effect is pre
those in the control group?

Answer 1

Fun. I prefer Oxymetazoline.

For the control group we have a headache probability of

c = 368/1671 = .220

For the experimental group we have a headache probability of

e = 494/2013 = .245

The observed difference is

d = e - c = .025

The variance of the difference is

s² = c(1-c)/n₁ + e(1-e)/n₂

so the standard deviation is

`s=√(.220(1-.220)/1671 + .245(1-.245)/2013) = 0.0139`

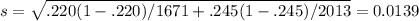
We get a t statistic on the difference of

t = d/s = .025/.0139 = 1.79

We're interested in the one sided test, P(d > 0). We have enough dfs to assume normality. We look up in the standard normal table

P(z < 1.79) = .96327

so

p = P(z > 1.79) = 1 - .96327 = 0.037 = 3.7%

Answer: That's less that 10% so we have evidence to conclude that headaches are significantly greater in the experimental group.