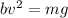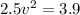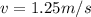Answer:
Part a)
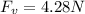
Part B)
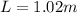
Part C)
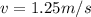
Step-by-step explanation:
Part A)
As we know that ball is hanging from the top and its angle with the vertical is 20 degree
so we will have
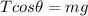

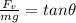
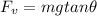

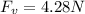
Part B)
Here we can use energy theorem to find the distance that it will move
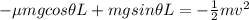
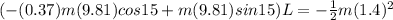
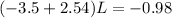
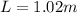
Part C)
At terminal speed condition we know that