Question

A farmer can plant a field in 10 days. His daughter can plant the same field in 15 days. If they work together how long would it take to plant the field?

Answer 1

The farmer and daughter working together would take 6 days to plant the field

Solution:

Time taken by farmer to plant a field is 10 days

Time taken by his daughter to plant a field is 15 days

Now lets find L.C.M of 10 and 15

List all prime factors for each number.

Prime factorization of 10 = 2 x 5

Prime factorization of 15 = 3 x 5

For each prime factor, find where it occurs most often as a factor and write it that many times in a new list.

The new superset list is

2, 3, 5

Multiply these factors together to find the LCM.

LCM = 2 x 3 x 5 = 30

Thus L.C.M of 10 and 15 is 30

`\begin{array}{l}{\text { Efficiency of farmer }=\frac{\text { Total Work }}{\text { Time Taken }}} \\\\ {\text { Efficiency of farmer }=(30)/(10)=3} \\\\ {\text { Efficiency of her daughter }=\frac{\text { Total Work }}{\text { Time Taken }}} \\\\ {\text { Efficiency of daughter }=(30)/(15)=2}\end{array}`

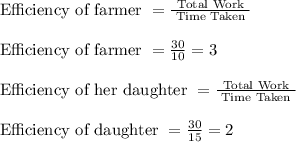
When both of them work together:

`\text { Time taken }=\frac{\text { Total work }}{\text { Total Efficiency }}=(30)/(3+2)=(30)/(5)=6 \text { days }`

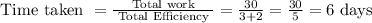
Hence, both of them can complete the work in 6 days