Answer:
 = 38.0° C
= 38.0° C
Step-by-step explanation:
The thermal transfer processes are governed by two equations one for there is a change of state, during this process the temperature does not change and another for which there is a change of temperature.
Q = ± m L
Q = m
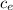 ΔT
ΔT
In calorimetric processes the heat transferred is equal to the heat absorbed by the system
-Qc = Qabs
Let's apply these equations to our system, we will assume the quantity A and B zero, reduce the magnitudes to the SI system
m1 = 10 g = 0.010 kg
m2 = 125 g = 0.125 kg
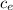 (ice) = 2090 J / kg ºC
(ice) = 2090 J / kg ºC
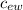 (water) = 4186 J / kg ºC
(water) = 4186 J / kg ºC
Lf = 3.33 105 J / kg
Let's write the expression for the heat given
Qc = m₂
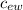 (T₀ -
(T₀ -
 )
)
Qc = 0.125 4186 (48.0 -
 )
)
Qc = 523.25 (48.0 -
 )
)
The expression for absorbed is
Qabs = Q1 + Q2 + Q3
calculate Q₁
Q₁ = m
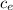 (
(
 - T₀)
- T₀)
Q₁ = 0.010 2090 (0 - (-15.0))
Q₁ = 313.5 J
Let's change state from ice to liquid water
Q₂ = + mL
Q₂ = 0.010 3.33 105
Q₂ = 3.33 103 J
Now let's heat the liquid water to the equilibrium temperature
Q₃ = m₁
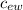 (
(
 -T₀)
-T₀)
Q₃ = 0.01 4186 (
 - 0)
- 0)
Q₃ = 41.86

let's calculate the maximum heat assigned
Qc = 0.125 4186 (48.0 - 0)
Qc = 2.5 104 J
Qc> (Q₂ + Q₁)
all the ice is melted
523.25 (48.0 -
 ) = Q1 + Q2 + 41.86
) = Q1 + Q2 + 41.86

(41.86 +523.25)
 = 523.25 48 - Q1 -Q2
= 523.25 48 - Q1 -Q2
565.11
 = 25116 - 313.5 - 3.33 103
= 25116 - 313.5 - 3.33 103
 = 21469.5 / 565.11
= 21469.5 / 565.11
 = 38.0 ° C
= 38.0 ° C