Question

A circular loop of wire with a radius of 12.0 cm and oriented in the horizontal xy-plane is located in a region of uniform magnetic field. A field of 1.6 T is directed along the positive z-direction, which is upward.A) If the loop is removed from the field region in a time interval of 2.8 ms, find the average emf that will be induced in the wire loop during the extraction process.E =??B)If the coil is viewed looking down on it from above, is the induced current in the loop clockwise or counterclockwise?

Answer 1

25.85081 V

Step-by-step explanation:

`\phi` = Magnetic flux

t = Time taken = 2.8 ms

B_i = Initial magnetic field

B_f = Final magnetic field

r = Radius of loop = 12 cm

A = Area =
`\pi r^2`

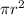
Induced emf is given by (Faraday's law)

`\epsilon=(\Delta\phi)/(\Delta t)\\\Rightarrow \epsilon=(B_fAcos90-B_iAcos0)/(\Delta t)\\\Rightarrow \epsilon=(B_iA)/(\Delta t)\\\Rightarrow \epsilon=(B\pi r^2)/(\Delta t)\\\Rightarrow \epsilon=(1.6* \pi 0.12^2)/(2.8* 10^(-3))\\\Rightarrow \epsilon=25.85081\ V`

The average emf that will be induced in the wire loop during the extraction process is 25.85081 V

Here the average emf induced is pointed toward z direction. If viewed from above then the direction of the current will be counter clockwise.