Question

For some transformation having kinetics that obey the Avrami equation (Equation 10.17), the parameter n is known to have a value of 2.1. If, after 146 s, the reaction is 50% complete, how long (total time) will it take the transformation to go to 86% completion?

Answer 1

t = 25.10 sec

Step-by-step explanation:

we know that Avrami equation

`Y = 1 - e^(-kt^n)`

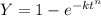
here Y is percentage of completion of reaction = 50%

t is duration of reaction = 146 sec

so,

`0.50 = 1 - e^(-k^146^2.1)`

`0.50 = e^(-k306.6)`

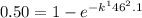
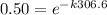
taking natural log on both side

ln(0.5) = -k(306.6)

`k = 2.26* 10^(-3)`

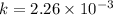
for 86 % completion

`0.86 = 1 - e^{-2.26* 10^(-3) * t^(2.1)}`

`e^{-2.26* 10^(-3) * t^(2.1)} = 0.14`

`-2.26* 10^(-3) * t^(2.1) = ln(0.14)`

`t^(2.1) = 869.96`

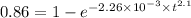
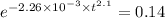
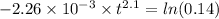
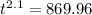
t = 25.10 sec