Question

Trouble finding the volume PLEASE HELP. Show work too

Answer 1

1697.41 cubic meters.

Explanation:

See the attached figure of the side triangle of the solid.

Let Δ ABC is the side triangle which is equilateral triangle, so AB = AC = BC = 14 m.

We have to find its area.

Let altitude AD divides BC into two equal parts BD = DC = 7 m

Now, Δ ADC is right triangle and AD² = AC² - DC² = 14² - 7² = 147

{Applying Pythagoras Theorem}

So, AD = 12.124 m

So, the area of the triangle is
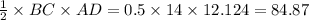`(1)/(2) * BC * AD = 0.5 * 14 * 12.124 = 84.87` sq. m.

Therefore, volume of the solid given in the figure is (84.87 × 20) = 1697.41 cubic meters. (Answer)