Question

A particle of mass 10 g and charge 72 μC moves through a uniform magnetic field, in a region where the free-fall acceleration is −9.8j m/s2. The velocity of the particle is a constant 19i hat km/s, which is perpendicular to the magnetic field. What, then, is the magnetic field? (Express your answer in vector form.)

Answer 1

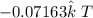`-0.07163\hat k\ T` or 0.07163 T into the page

Step-by-step explanation:

m = Mass of particle = 10 g

a = Acceleration due to gravity = -9.8j m/s²

v = Velocity of particle = 19i km/s

q = Charge of particle = 72 μC

B = Magnetic field

Here the magnetic and gravitational forces on the particle are applied in the opposite direction so,

`F_b=F_g`

`F_b=qvBsin\theta\\\Rightarrow F_b=qvBsin90\\\Rightarrow F_b=72* 10^(-6)* 19000B`

`F_g=ma\\\Rightarrow F_g=0.01* -9.8`

`72* 10^(-6)* 19000B=0.01* -9.8\\\Rightarrow B=(0.01* -9.8)/(72* 10^(-6)* 19000)\\\Rightarrow B=-0.07163\hat k\ T`

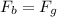
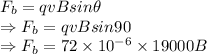
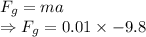
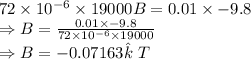
The magnetic field is 0.07163 T into the page