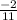Question

25 point for this question:

For which values of a and b the following is true for any real number x?

`(x-4)/x^2+7x-18=a/x+9+b/x-2`

Answer 1

a=
`(13)/(11)` b =
`(-2)/(11)`

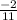
Explanation:

• By equating coefficients on both sides of respective terms.

Given,

`(x-4)/(x^2+7x-18)` =
`(a)/(x+9)` +
`(b)/(x-2)`

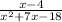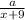Now take lcm on right hand side

`(x-4)/(x^2+7x-18)` =
`(a(x-2)+b(x+9))/(x^2+7x-18)`

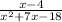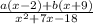
By equating coefficients of respective terms

x-4 = a(x-2)+b(x+9)

a+b=1 -----1 ; 9b-2a=-4 -----2

Substitute b=1-a in 2

9(1-a)-2a=-4

11a=9+4=13

a=
`(13)/(11)`

As b=1-a=1-
`(13)/(11)`=
`(-2)/(11)`