Question

The coil in a generator has 100 windings and a cross sectional area of 0.01 m^2. If the coil rotates with constant speed in the magnetic field of the earth (B = 0.5x10^-4 T), how many revolutions per second must it complete to generate a maximum e.m.f. of one volt?

Using this result, is it practical to create a generator that uses only the earths magnetic field?

Answer 1

To develop the problem it is necessary to apply the concepts related to the induced voltage and its definition according to the magnetic field and angular velocity.

By definition the induced voltage or electromotive force is given by

`\epsilon = BAN\omega`

Where,

B = Magnetic Field

A = Cross-sectional area

N = Number of turns

`\omega`= Angular velocity

For the given case in the problem we will look for the angular velocity,

`\omega = (\epsilon)/(BAN)\\\omega = (1)/((0.5*10^(-4))(0.01)(100))\\\omega = 2*10^4rad/s \\\omega = 2*10^4rad/s ((1rev)/(2\pi rad))\\\omega = 3183.098rev/s`

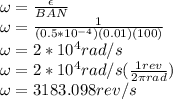
Therefore the number of revolutions is 3183.1rev/s.

Although the number of revolutions the magnetic field of the earth is discarded for use as a generator because the magnetic field of the earth compared to other current devices is considered weak. Very little power would be obtained from the generator