Question

A steel ball, with diameter = 3 mm and density = 7600 kg/m3 is dropped in a liquid with density = 1200 kg/m3 . The average time for the ball to travel a distance of 0.50 m is 10.0 s. a. Does the ball rise or fall in the fluid? Explain b. Determine the fluid viscosity.

Answer 1

given,

diameter of the steel ball = 3 m = 0.003 m

density of the steel = 7600 kg/m³

density of liquid = 1200 kg/m³

distance travel by the ball = 0.5 m

time = t = 10 s

average velocity =
`v = (0.5)/(10)`

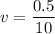
v = 0.05 m/s

a) density of water is less than ball so, ball will fall in the fluid.

gravitational force is equal to buoyancy force and the drag force

`F_g = F_b + F_d`

`F_g = \rho_g Vg`

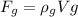
Density of ball = ρ_s

V is the volume ball

buoyancy force

`F_b = \rho_f Vg`

`F_b = \rho_f V g`

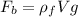
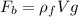
drag force

`F_d =3 \pi \mu d v`

`F_g = F_b + F_d`

`\rho_g Vg = \rho_f V g+ 3 \pi \mu d v`

`(\rho_g - \rho_f)Vg = 3 \pi \mu d v`

`V= (1)/(6)\pi d^3`

`(\rho_g - \rho_f). (1)/(6)\pi d^3.g = 3 \pi \mu d v`

`\mu = ((\rho_s-\rho_f)d^2g)/(18 v)`

`\mu = ((7600 -1200)* 0.003^2* 9.8 )/(18 * 0.05)`

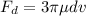
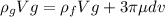
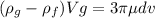
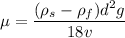
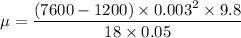
μ = 0.63 kg m/s