Answer:
The function g(x) = - x² + 14x + 39 is at a minimum when x = 7 is not true i.e. false.
Explanation:
The function is given to be g(x) = - x² + 14x + 39 .......... (1)
Now, the condition for maxima or minima is
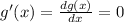 .
.
Now, differentiating equation (1) we get, g'(x) = - 2x + 14 ........ (2)
Hence, for maxima or minima g'(x) = 0 = - 2x + 14
⇒ x = 7
Now, from equation (2) and differentiating both sides with respect to x again
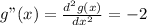 < 0
< 0
Therefore,the function g(x) has maxima at x = 7
Therefore, the function g(x) = - x² + 14x + 39 is at a minimum when x = 7 is not true i.e. false.