Question

One object (m1 = 0.220 kg) is moving to the right with a speed of 2.10 m/s when it is struck from behind by another object (m2 = 0.345 kg) that is moving to the right at 6.00 m/s. If friction is negligible and the collision between these objects is elastic, find the final velocity of each. (Indicate the direction with the sign of your answer. Positive is to the right, and negative is to the left.)

Answer 1

vf₁ = 6.86 m/s , to the right

vf₂ = 2.96 m/s, to the right

Step-by-step explanation:

Theory of collisions

Linear momentum is a vector magnitude (same direction of the velocity) and its magnitude is calculated like this:

p=m*v

where

p:Linear momentum

m: mass

v:velocity

There are 3 cases of collisions : elastic, inelastic and plastic.

For the three cases the total linear momentum quantity is conserved:

P₀ = Pf Formula (1)

P₀ :Initial linear momentum quantity

Pf : Final linear momentum quantity

Data

m₁= 0.220 kg : mass of object₁

m₂= 0.345 kg : mass of object₂

v₀₁ = 2.1 m/s ₁ , to the right : initial velocity of m₁

v₀₂= 6 m/s, to the right i :initial velocity of m₂

Problem development

We appy the formula (1):

P₀ = Pf

m₁*v₀₁ + m₂*v₀₂ = m₁*vf₁ + m₂*vf₂

We assume that the two objects move to the right at the end of the collision, so, the sign of the final speeds is positive:

(0.22)*(2.1) + (0.345)*(6) = (0.22)*vf₁ +(0.345)*vf₂

2.532 = (0.22)*vf₁ +(0.345)*vf₂ Equation (1)

Because the shock is elastic, the coefficient of elastic restitution (e) is equal to 1.

`e= (v_(f2)-v_(f1) )/(v_(o1) -v_(o2) )`

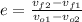
1*(v₀₁ - v₀₂ ) = (vf₂ -vf₁)

(2.1 - 6 ) = (vf₂ -vf₁)

-3.9 = (vf₂ -vf₁)

vf₂ = vf₁ - 3.9

vf₂ = vf₁ - 3.9 Equation (2)

We replace Equation (2) in the Equation (1)

2.532 = (0.22)*vf₁ +(0.345)*( vf₁ - 3.9)

2.532 = (0.22)*vf₁ +(0.345)* (vf₁) -(0.345)( 3.9)

2.532 + 1.3455 = (0.565)*vf₁

3.8775 = (0.565)*vf₁

vf₁ = (3.8775) / (0.565)

vf₁ = 6.86 m/s, to the right

We replace vf₁ = 6.86 m/s in the Equation (2)

vf₂ = 6.86 - 3.9

vf₂ = 2.96 m/s, to the right