Question

A student sits at rest on a piano stool that can rotate without friction. The moment of inertia of the student-stool system is 4.4 kg⋅m2 . A second student tosses a 2.0 kg mass with a speed of 3.0 m/s to the student on the stool, who catches it at a distance of 0.45 m from the axis of rotation.

Answer 1

0.56191 rad/s

Step-by-step explanation:

r = Distance at which the ball is caught = 0.45 m

m = Mass of ball = 2 kg

v = Velocity of ball = 3 m/s

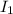`I_1` = Moment of inertia of stool student system = 4.4 kgm²

Initial angular momentum is

`L_i=mvr`

As the stool rotates the linear momentum is not conserved but the angular momentum is conserved.

Final angular momentum is

`L_f=I\omega`

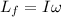
Moment of inertia is given by

`I=I_1+mr^2`

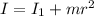
`mr^2` = Moment of inertia of ball

As the angular momentum of the system is conserved

`L_i=L_f\\\Rightarrow mvr=(I_1+mr^2)\omega\\\Rightarrow \omega=(mvr)/(I_1+mr^2)\\\Rightarrow \omega=(2* 3* 0.45)/(4.4+2* 0.45^2)\\\Rightarrow \omega=0.56191\ rad/s`

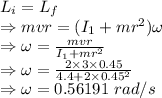
The angular speed of the stool is 0.56191 rad/s