Question

The angular momentum of a flywheel having a rotational inertia of 0.142 kg·m2 about its central axis decreases from 7.20 to 0.140 kg·m2/s in 2.10 s. (a) What is the magnitude of the average torque acting on the flywheel about its central axis during this period? (b) Assuming a constant angular acceleration, through what angle does the flywheel turn? (c) How much work is done on the wheel? (d) What is the magnitude of the average power done on the flywheel?

Answer 1

3.3619 Nm

54.27472 rad

182.46618 J

86.88 W

Step-by-step explanation:

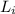`L_i` = Initial angular momentum = 7.2 kgm²/s

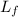`L_f` = Final angular momentum = 0.14 kgm²/s

I = Moment of inertia = 0.142 kgm²

t = Time taken

Average torque is given by

`\tau_(av)=(L_f-L_i)/(\Delta t)\\\Rightarrow \tau_(av)=(0.14-7.2)/(2.1)\\\Rightarrow \tau_(av)=-3.3619\ Nm`

Magnitude of the average torque acting on the flywheel is 3.3619 Nm

Angular speed is given by

`\omega_i=(L_i)/(I)`

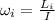
Angular acceleration is given by

`\alpha=(\tau)/(I)`

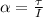
From the equation of rotational motion

`\theta=\omega_it+(1)/(2)\alpha t^2\\\Rightarrow \theta=(L_i)/(I)* t+(1)/(2)* (\tau)/(I)* t^2\\\Rightarrow \theta=(7.2)/(0.142)* 2.1+(1)/(2)* (-3.3619)/(0.142)* 2.1^2\\\Rightarrow \theta=54.27472\ rad`

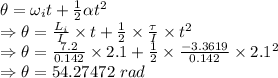
The angle the flywheel turns is 54.27472 rad

Work done is given by

`W=\tau\theta\\\Rightarrow W=-3.3619* 54.27472\\\Rightarrow W=-182.46618\ J`

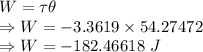
Work done on the wheel is 182.46618 J

Power is given by

`P=(W)/(t)\\\Rightarrow P=(-182.46618)/(2.1)\\\Rightarrow P=-86.88\ W`

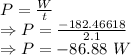
The magnitude of the average power done on the flywheel is 86.88 W