Answer:
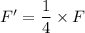
Step-by-step explanation:
The electric force between two charged particle is given by :
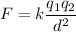
d is the distance between charged particles
Let F' is the force when the distance between the particles is doubled, d' = 2d
New force is given by :
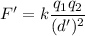
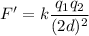
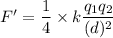
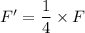
The new force becomes one fourth of the original force. So, if the distance between the particles is doubled, the force becomes one fourth.