Answer: Option 'd' is correct.
Explanation:
Since we have given that
Researcher A :
Mean = 12.4
Standard deviation = 9
sample size = 30
So, the standard error is given by

Researcher B:
Mean = 12.4
Standard deviation = 9
Sample size = 40
So, the standard error is given by
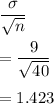
Sample B has smaller standard error than sample A because the sample size was larger than A.
Hence, Option 'd' is correct.