Answer:
1.96
Explanation:
Solution:-
- We will use the table given to determine the standard deviation of random variable x. From descriptive statistics we have the following formula for standard deviation (s.d) :
s.d (x) = sqrt ( Var(x) )
- The formula for Variance ( Var (x) ) is also taken from descriptive statistics as follows:
![Var(X) = E ( X^2) + [ E(X) ] ^2](https://img.qammunity.org/2020/formulas/mathematics/high-school/xkmr8u37dkbzilgiw9gyfdm0knq4nxw3ez.png)
- Where,
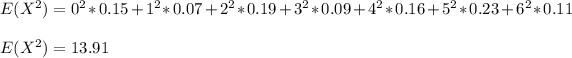
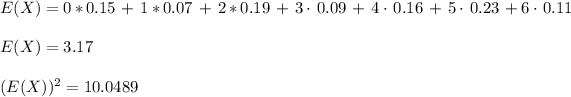
- So the variance is:
Var ( X ) = 13.91 - 10.0489 = 3.8611
s.d (x) = √3.8611 = 1.96