Question

Suppose that Stephen is the quality control supervisor for a food distribution company. A shipment containing many thousands of apples has just arrived. Unknown to Stephen, 15% of the apples are damaged due to bruising, worms, or other defects. If Stephen samples 10 apples from the shipment, use the binomial distribution to estimate the probability that his sample will contain at least one damaged apple. Give your answer as a decimal precise to at least four decimal places.

Answer 1

Answer: 0.8031

Explanation:

Binomial distribution:

For a binomial variable x,

The probability of getting success in x trials =
`P(X=x)=^nC_xp^x(1-p)^(n-x)`

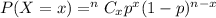
, where n = Total trials .

p= Probability of getting success in each trial.

For the given situation , we take x as the number of damaged apple .

Given : The proportion of damaged apples : p=0.15

n= 10

Then, the probability that his sample will contain at least one damaged apple. :-

`P(x\geq1)=1-P(x<1)\\\\=1-P(x=0)\\\\=1-^(10)C_(0)(0.15)^0(1-0.15)^(10)\\\\=1-(1)(0.85)^(10)\ \ [\because\ ^nC_0=1]\\=1-0.196874404341=0.803125595659\approx0.8031`

Hence, the required probability = 0.8031