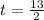Question

The height (feet) of an object moving vertically is given by s= (-16t^2)+(208t)+(156), where t is in seconds.

Find the objects velocity at t=7, its maxumum height and when it occurs, and its velocity.

Answer 1

385

Explanation:

Answer 2

The object's velocity at t = 7 is -16 ft/s

The maximum height is 832 ft and it occurs when
`t=(13)/(2)`

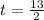
Explanation:

From the information given, the equation of motion of the object is

`s(t)= -16t^2+208t+156`

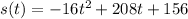
For any equation of motion s(t), the instantaneous velocity at time t is given by

`v(t)=(ds)/(dt)`

(a) To find the object's velocity at t = 7, you must:

`v(t)=(d)/(dt)(-16t^2+208t+156)\\\\v(t)=-(d)/(dt)\left(16t^2\right)+(d)/(dt)\left(208t\right)+(d)/(dt)\left(156\right)\\\\v(t)=-32t+208+0\\\\v(t)=-32t+208`

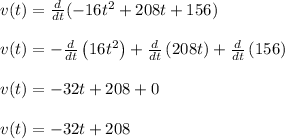
Next, we evaluate when t = 7

`v(7)=-32(7)+208=-224+208\\\\v(7)=-16`

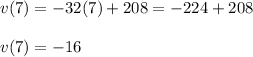
The object's velocity at t = 7 is -16 ft/s

(b) To find the maximum of a function we always use the derivative of the function and we set it equal zero to find the critical points.

To find the maximum height and when it occurs, we set the velocity function equal to 0 and solve for t.

`-32t+208=0\\\\-32t+208-208=0-208\\\\-32t=-208\\\\(-32t)/(-32)=(-208)/(-32)\\\\t=(13)/(2)`

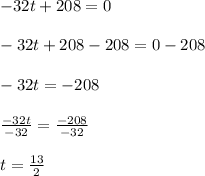
Next, we substitute this value into the equation of motion to find the maximum height

`s((13)/(2))= -16((13)/(2))^2+208((13)/(2))+156\\\\s((13)/(2))=-676+1352+156)=832`

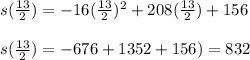
The maximum height is 832 ft and it occurs when
`t=(13)/(2)`