Question

Find the rational zeros of the polynomial function, f(x)= 4x^3-8x^2-19x-7

Answer 1

The rational zero of the polynomial are
`\pm (7)/(4), \pm (1)/(4),\pm (7)/(2),\pm (1)/(2),\pm 7,\pm 1` .

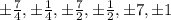Explanation:

Given polynomial as :

f(x) = 4 x³ - 8 x² - 19 x - 7

Now the ration zero can be find as

`(\textrm factor of P)/(\textrm factor Q)` ,

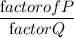where P is the constant term

And Q is the coefficient of the highest polynomial

So, From given polynomial , P = -7 , Q = 4

Now ,
`(\textrm factor of \pm P)/(\textrm factor of \pm Q)`

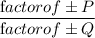
I.e
`(\textrm factor of \pm P)/(\textrm factor of \pm Q)` =
`(\pm 7 , \pm 1)/(\pm 4 ,\pm 2,\pm 1 )`

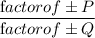Or, The rational zero are
`\pm (7)/(4), \pm (1)/(4),\pm (7)/(2),\pm (1)/(2),\pm 7,\pm 1`

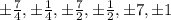
Hence The rational zero of the polynomial are
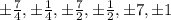`\pm (7)/(4), \pm (1)/(4),\pm (7)/(2),\pm (1)/(2),\pm 7,\pm 1` . Answer