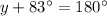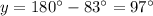Question

1. In triangle XYZ, A is the midpoint of XY, B is the midpoint of YZ, and C is the midpoint of XZ. Also, AY = 7, BZ = 8, and XZ = 18. What is the perimeter of triangle ABC? (SHOW WORK)

2. What is y? (SHOW WORK) 2nd picture is the triangle.

Answer 1

Part 1) The perimeter of triangle ABC is 24 units

Part 2)
`y=97\°`

Explanation:

Part 1)

we know that

The Midpoint Theorem states that the segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side

The perimeter of triangle ABC is equal to

`P=AB+BC+AC`

Applying the Midpoint Theorem

Find the measure of AB

`AB=(XZ)/(2)`

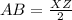
substitute given value

`AB=(18)/(2)=9\ units`

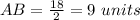
Find the measure of BC

`BC=(XY)/(2)`

`XY=2AY`

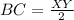
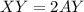
substitute given value

`XY=2(7)=14\ units`

`BC=(14)/(2)=7\ units`

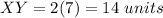
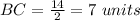
Find the measure of AC

`AC=(YZ)/(2)`

`YZ=2BZ`

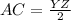
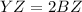
substitute given value

`YZ=2(8)=16\ units`

`AC=(16)/(2)=8\ units`

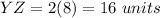
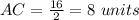
Find the perimeter of triangle ABC

`P=9+7+8=24\ units`

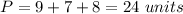
Part 2)

step 1

Find the measure of angle z

Remember that the sum of the interior angles in a triangle must be equal to 180 degrees

`55\°+42\°+z=180\°\\97\°+z=180\°\\z=180\°-97\°\\z=83\°`

step 2

Find the measure of angle y

we know that

`y+z=180\°` ----> by supplementary angles (form a linear pair)

substitute the value of z

`y+83\°=180\°`

`y=180\°-83\°=97\°`