Answer:
It's expected to win $17 in this game
Explanation:
The expected value for a probability distribution is given by
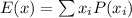
In this problem, the set of x is
x={$56 , $36 , -$52}
Which have the respective set of probabilities:
p(x)={0.37 , 0.33 , 1-0.37-0.33 }={ 0.37 , 0.33 , 0.3 }
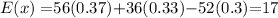
It's expected to win $17 in this game