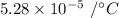Question

At 16°C, a rod is exactly 23.59 cm long on a steel ruler. Both the rod and the ruler are placed in an oven at 260°C, where the rod now measures 23.83 cm on the same ruler. What is the coefficient of thermal expansion for the material of which the rod is made? The linear expansion coefficient of steel is 11 x 10-6 /C°.

Answer 1

`5.28* 10^(-5)\ /^(\circ)C`

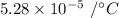
Step-by-step explanation:

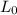`L_0` = Original length of rod

`\alpha` = Coefficient of linear expansion =
`1.62* 10^(-5)\ /^(\circ)C`

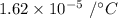
Initial temperature = 16°C

Final temperature = 260°C

Change in length of a Steel is given by

`\Delta L=\alpha L_0\Delta T\\\Rightarrow \Delta L=11* 10^(-6)* 23.83* (260-16)\\\Rightarrow \Delta L=0.06395972\ cm`

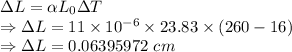
Change in material rod length will be

`23.83-23.59+0.0639572=0.3039572\ cm`

The coefficient of thermal expansion is given by

`\alpha=(\Delta L)/(L_0\Delta T)\\\Rightarrow \alpha=(0.3039572)/(23.59* (260-16))\\\Rightarrow \alpha=5.28* 10^(-5)\ /^(\circ)C`

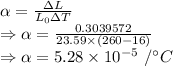
The coefficient of thermal expansion for the material is
`5.28* 10^(-5)\ /^(\circ)C`