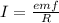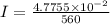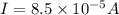Question

A coil 3.95 cm radius, containing 520 turns, is placed in a uniform magnetic field that varies with time according to B=( 1.20×10−2 T/s )t+( 3.45×10−5 T/s4 )t4. The coil is connected to a 560-Ω resistor, and its plane is perpendicular to the magnetic field. You can ignore the resistance of the coil.

a-)Find the magnitude of the induced emf in the coil as a function of time.

Find the magnitude of the induced emf in the coil as a function of time.

E= 1.07×10−2 V +( 1.05×10−4 V/s3 )t3
E= 3.36×10−2 V +( 8.26×10−5 V/s3 )t3
E= 3.36×10−2 V +( 3.30×10−4 V/s3 )t3
E= 1.07×10−2 V +( 3.30×10−4 V/s3 )t3
b-)What is the current in the resistor at time t0 = 5.25 s ?

Answer 1

(a) E= 3.36×10−2 V +( 3.30×10−4 V/s3 )t3

(b)
`I=0.0085\ A`

Step-by-step explanation:

Given:

• radius if the coil,
  `r=0.0395\ m`

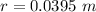
• no. of turns in the coil,
  `n=520`

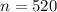
• variation of the magnetic field in the coil,
  `B=(1.2* 10^(-2))t+(3.45* 10^(-5))t^4`

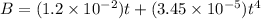
• resistor connected to the coil,
  `R=560\ \Omega`

(a)

we know, according to Faraday's Law:

`emf=n.(d\phi)/(dt)`

where:

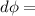`d \phi=` change in associated magnetic flux

`\phi= B.A`

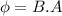
where:

A= area enclosed by the coil

Here

`A=\pi.r^2`

`A=\pi* 0.0395^2`

`A=0.0049\ m^2`

`\therefore \phi=((1.2* 10^(-2))t+(3.45* 10^(-5))t^4)* 0.0049`

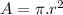
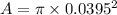
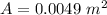
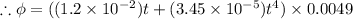
So, emf:

`emf= 520* (d)/(dt) [((1.2* 10^(-2))t+(3.45* 10^(-5))t^4)* 0.0049]`

`emf= 520* 0.0049* (d)/(dt) [(1.2* 10^(-2))t+(3.45* 10^(-5))t^4)]`

`emf= 2.548* [0.012+(13.8* 10^(-5))t^3)]`

`emf= 0.0306+3.516* 10^(-4)\ t^3`

(b)

Given:

`t_0=5.25\ s`

Now, emf at given time:

`emf=4.7755* 10^(-2)\ V`

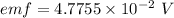
∴Current

`I=(emf)/(R)`

`I=(4.7755* 10^(-2))/(560)`

`I=8.5* 10^(-5) A`