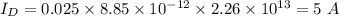Question

Two circular plates of radius 9cm are separated in air by 2.0mm, forming a parallel plate capacitor. A battery is connected across the plates. At a particular time, t1, the rate at which the charge is flowing through the battery from one plate to the other is 5A. (a)What is the time rate of change of the electric field between the plates at t1? (b)Compute the displacement current between the plates at t1, and show it is equal to 5A.

Answer 1

(a)
`2.26* 10^(13)\ N/C.s`

(b) 5 A

Solution:

As per the question:

Radius of the circular plate, R = 9 cm = 0.09 m

Distance, d = 2.0 mm =
`2.0* 10^(- 3)\ m`

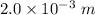
At
`t_(1)`, current, I = 5 A

Now,

Area, A =
`\pi R^(2) = \pi 0.09^(2) = 0.025`

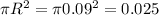
We know that the capacitance of the parallel plate capacitor, C =
`(\epsilon_(o) A)/(d)`

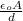
Also,

`q = CV`

`q = (\epsilon A)/(d)V`

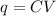
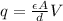
Also,

`V = (E)/(d)`

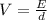
Now,

(a) The rate of change of electric field:

`(dE)/(dt) = (dq)/(dt)((1)/(A\epsilon_(o)))`

where

`I = (dq)/(dt) = 5\ A`

`(dE)/(dt) = 5* ((1)/(0.025* 8.85* 10^(- 12))) = 2.26* 10^(13)\ N/C.s`

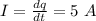
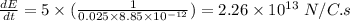
(b) To calculate the displacement current:

`I_(D) = epsilon_(o)* (d\phi)/(dt)`

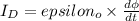
where

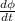`(d\phi)/(dt)` = Rate of change of flux

`I_(D) = Aepsilon_(o)* (dE)/(dt)`

`I_(D) = 0.025* 8.85* 10^(- 12)* 2.26* 10^(13) = 5\ A`