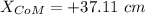Answer:
The center of mass is at + 37.11 cm
Solution:
As per the question:
Length of the rod, l = 1 m = 100 cm
Density of Brass,
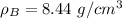
Density of Aluminium,
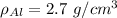
Distance from the mid point to each, r = 50 cm
Now,
The center of mass with the origin at mid point is given by the expression:
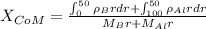
![X_(CoM) = (\rho_(B)(r^(2))/(2)]_(0)^(50) + \rho_(Al)(r^(2))/(2)]_(100)^(50))/(M_(B)r + M_(Al)r)](https://img.qammunity.org/2020/formulas/physics/college/j51qndp9crb1gfhzw47emwczhntondwkhi.png)
![X_(CoM) = \frac{8.44(2500)/(2)] + 2.7(7500)/(2)]{8.44* 50 + 2.7* 50}]()