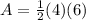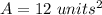Question

on the coordinate plane, draw a triangle ABC with vertices A(-3,3),B(-3,-3), C(1,-3), find the area of the triangle in square units

Answer 1

The area of triangle is 12 square units

Explanation:

we have

The triangle ABC with vertices A(-3,3),B(-3,-3), C(1,-3)

step 1

Using a graphing tool

Plot the triangle

see the attached figure

The triangle ABC is a right triangle

step 2

Find the area of triangle ABC

The area of the triangle ABC is equal to

`A=(1)/(2)(BC)(AB)`

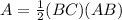
we have

`BC=1-(-3)=4\ units` ---> the distance is the difference of the x-coordinates

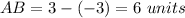`AB=3-(-3)=6\ units` ---> the distance is the difference of the y-coordinates

substitute the values

`A=(1)/(2)(4)(6)`

`A=12\ units^2`