Question

The bacterial strain Acinetobacter has been tested for its adhesion properties, which is believed to follow a normal distribution. A sample of five measurements gave readings of 2.69, 5.76, 2.67, 1.62 and 4.12 dyne-cm. Assume that the standard deviation is known to be 0.7 dyne-cm_2 and that the scientists are interested in high adhesion (at least 2.66 dyne-cm^2)(a) Should the alternative hypothesis be one-sided or two-sided? Write down the null and alternative hypotheses.(b) Based on your answer to part (a), test the hypothesis to see if the mean adhesion is at least 2.66 dyne-cm_2 (Use the p-value approach) What can be concluded?

Answer 1

a) Alternative hypothesis should be one sided. Because Null and Alternative hypotheses are:

`H_(0)`: μ=2.66 dyne-cm.

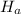`H_(a)`: μ<2.66 dyne-cm.

b) the hypothesis that mean adhesion is at least 2.66 dyne-cm is true

Explanation:

Let μ be the mean adhesion in dyne-cm.

a)

Null and alternative hypotheses are:

`H_(0)`: μ=2.66 dyne-cm.

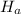`H_(a)`: μ<2.66 dyne-cm.

b)

First we need to calculate test statistic and then the p-value of it.

test statistic of sample mean can be calculated as follows:

t=
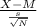`(X-M)/((s)/(√(N) ) )` where

• X is the sample mean

• M is the mean adhesion assumed under null hypothesis (2.66 dyne-cm)

• s is the standard deviation known (0.7 dyne-cm_2)

• N is the sample size(5)

Sample mean is the average of 2.69, 5.76, 2.67, 1.62 and 4.12 dyne-cm, that is
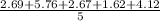`(2.69+5.76+2.67+1.62+4.12)/(5)` ≈ 3.37

using the numbers we get

t=
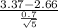`(3.37-2.66)/((0.7)/(√(5) ) )` ≈ 2.27

The p-value is ≈ 0.043. Taking significance level as 0.05, we can conlude that sample proportion is significantly higher than 2.66 dyne-cm.

Thus, according to the sample the hypothesis that mean adhesion is at least 2.66 dyne-cm is true