Answer:
-25 rad/s²
29 times
3.8 seconds
50.54 m
26.6 m/s
Step-by-step explanation:
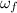 = Final angular velocity
= Final angular velocity
 = Initial angular velocity
= Initial angular velocity
 = Angular acceleration
= Angular acceleration
 = Angle of rotation
= Angle of rotation
a = Acceleration = -7 m/s² (negative because of deceleration)
r = Radius of wheel = 0.28 m
Angular acceleration is given by

Angular acceleration of the wheel is -25 rad/s²
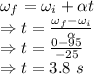
It took 3.8 seconds for the car to stop
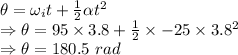
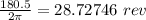
The wheel rotated 29 times.
The
Initial velocity
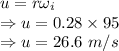
The car’s initial velocity is 26.6 m/s
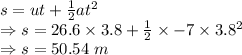
Distance covered in the time is 50.54 m
If the distance is found in meters we get 50.54 m which is very low. Considering the initial velocity is 95.76 km/h. Coming to a stop in that distance is very low. So, the values do not seem reasonable. At least a 100 m is required to stop from that speed.