Answer:
(a). The change in the protons electric potential is 0.639 kV.
(b). The change in the potential energy of the proton is
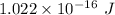
(c). The work done on the proton is
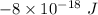 .
.
Step-by-step explanation:
Given that,
Speed

Initial potential V=100 V
Final potential = 150 V
(a). We need to calculate the change in the protons electric potential
Potential energy of the proton is

Using conservation of energy

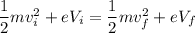
/(2)mv_(i)^2-](1)/(2)mv_(f)^2=e(V_(f)-V_(i))](https://img.qammunity.org/2020/formulas/physics/college/8y1gtca4hks06r64a5rqpotxsi0he0h83k.png)
/(2)mv_(f)^2=e\Delta V](https://img.qammunity.org/2020/formulas/physics/college/5jlrxwwykaxaiw29gwrq02g32hnlhnhcsr.png)
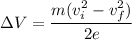
Put the value into the formula
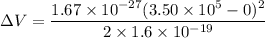
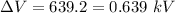
(b). We need to calculate the change in the potential energy of the proton
Using formula of potential energy
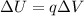
Put the value into the formula
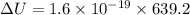
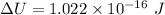
(c). We need to calculate the work done on the proton
Using formula of work done

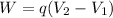


Hence, (a). The change in the protons electric potential is 0.639 kV.
(b). The change in the potential energy of the proton is
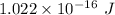
(c). The work done on the proton is
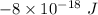 .
.