Question

Which of the following represents the graph below? See attachment ​

Answer 1

9514 1404 393

Answer:

f(x) = (x -a)²(x -b)⁴

Explanation:

The function shown is of even degree (both ends tend in the same direction), so choices 1 and 3 are eliminated.

The graph does not cross the x-axis at either of the zeros. This means the zeros must have even multiplicity. The only viable choice* is the second one:

`f(x)=(x-a)^2(x-b)^4`

_____

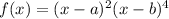
* Additional comment

In practice, the curve shown will be generated by a function of the form ...

f(x) = c(x -a)²(x -b)²

where the powers of the zeros are exactly 2, not just any even number. For higher multiplicity, the curve is increasingly "flat" at the zero. For different multiplicities, the curve is asymmetrical about the y-axis. The attachment shows the shape for exponents of 2 and 4 as in this problem statement. (That is, none of the offered answer choices is correct.)

If the grid shown in the problem statement is square (same scaling in x- and y-directions), the values of a and b must be near 1, and each grid square must represent a fraction of a unit, near 1/4. In the second attachment, the grid squares are about 0.273 units each.