Question

A large, cylindrical water tank with diameter 3.60 m is on a platform 2.00 m above the ground. The vertical tank is open to the air and the depth of the water in the tank is 2.00 m. There is a hole with diameter 0.520 cm in the side of the tank just above the bottom of the tank. The hole is plugged with a cork. You remove the cork and collect in a bucket the water that flows out the hole. Part A When 1.00 gal of water flows out of the tank, what is the change in the height of the water in the tank?

Answer 1

To solve this problem it is necessary to apply the concepts related to the geometry of a cylindrical tank and its respective definition.

The volume of a tank is given by

`V = (\pi d^2)/(4)h`

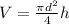
Where

d = Diameter

h = Height

Considering that there are two stages, let's define the initial and final volume as,

`V_0 = (\pi d^2)/(4)H`

`V_f = (\pi d^2)/(4)h`

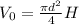
We know as well by definition that

`1gal = 3.785*10^(-3)m^3`

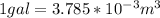
Then we have for the statement that

`V_f = V_0 -1gal`

`V_f = V_0 - 3.785*10^(-3)`

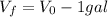
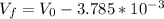
Replacing the previous data

`(\pi d^2)/(4)h = (\pi d^2)/(4)H- 3.785*10^(-3)`

`(\pi (3.6)^2)/(4)h = (\pi (3.6)^2)/(4)(2)- 3.785*10^(-3)`

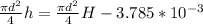
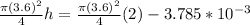
Solving to get h,

`h = 1.99963m`

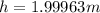
Therefore the change is

`\Delta h = H-h`

`\Delta h = 2- 1.99963`

`\Delta h = 3.7*10^(-4)m=0.37mm`

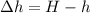
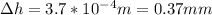
Therefore te change in the height of the water in the tank is 0.37mm