Question

5.

The present value of a sum of money is the
amount that must be invested now, at a given
rate of interest, to produce the desired sum at a
later date. Find the present value of 10,000 if
interest is paid at a rate of 6.2% compounded
weekly for 8 years.

Answer 1

The present value of 10,000 if interest is paid at a rate of 6.2% compounded weekly for 8 years is 6097.56

Step-by-step explanation:

We know that compound interest is given by

`A=P\left(1+(r)/(n)\right)^(n t)`

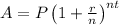
Where ,

Where A = final amount (which is given to be = 10000)

P = Principal amount (which is the present amount which we have to find)

r = interest rate = 6.2 = 0.062

n = no. of times interest applied per time period = it is given that the interest is applied weekly, so in one year there are 52 weeks so n = 52

t = time period = 8 years

Substituting the given values, we get

`10000=\mathrm{P}\left(1+(6.2)/(52)\right)^(52* 8)`

P = 6097.5

We get, P = 6097.56 which is the present value of a sum of money