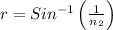Question

A ray of light in water (index n1) is traveling upward toward the air and is incident at the water-air boundary at the critical angle. Some oil (index n2) is now floated on the water. The angle between the direction of the ray in the oil and the normal direction is:A. sin-1(1/n1)B. sin-1(1/n2)C. sin-1(n1/n2)D. sin-1(1.00)E. sin-1(n2/n1)

Answer 1

`r = Sin^(-1)\left ( (1)/(n_(2)) \right )`

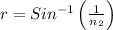
Option (B) is true

Step-by-step explanation:

refractive index of water = n1

refractive index of air = na = 1

refractive index of oil = n2

When the ray goes from water to air

Use Snell's law

Let the angle of incidence is i

n1 Sin i = na x Sin r

For total internal reflection, r = 90°

n1 x Sin i = 1 x Sin 90

Sin i = 1 / n1 .... (1)

For water oil interface

angle of incidence is i and let the angle of refraction is r.

n1 x Sin i = n2 x Sin r

n1 x / 1 n1 = n2 Sin r (from equation (1)

Sinr = 1/n2

`r = Sin^(-1)\left ( (1)/(n_(2)) \right )`