Question

Four point masses, each of mass 1.3 kg are placed at the corners of a rigid massles square of side 1.1 m. Find the moment of inertia of this system about an axis that is perpendicular to the plane of the square and passes through one of the masses.

Answer 1

I= 6.292 kg.m²

Step-by-step explanation:

Given that

m = 1.3 kg

Side of square a= 1.1 m

The distance r

`r=\sqrt{{a^2}+{a^2}}`

`r={a}{\sqrt 2}`

`r={1.1}{\sqrt 2}`

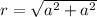
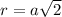
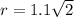
The moment of inertia I

The axis passes through one of the mass then the distance of the that mass from the axis will be zero.

I = m a² + m a² + m r²

By putting the values

I = m a² + m a² + m r²

I =m( 2 a² + r²)

I =1.3( 2 x 1.1² + 2 x 1.1²)

I = 1.3 x 4 x 1.1² kg.m²

I= 6.292 kg.m²