Question

Farmer bob's square plot ofland is slowly eroding away. Worried about the future of his farm. Farmer Bob measures the rate of erosion and finds that the length of each side of his square plot is decreasing at the constant rate of 2 feet/year. If he currently owns 250,000 square feet of land, what is the current rate of change of the area of Farmer Bob's land?

a) Farmer bob is losing 2,000 square feet of land per year
b) losing 1,000,000 square feet of land per year
c) losing 1,000 square feet of land per year
d) losing 4 square feet of land per year

Answer 1

Option A.

Explanation:

Area of a square is

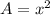`A=x^2` .... (1)

where, x is side length.

The length of each side of his square plot is decreasing at the constant rate of 2 feet/year.

`(dx)/(dt)=2`

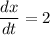
It is given that bob currently owns 250,000 square feet of land.

Fist find the length of each side.

`A=250000`

`x^2=250000`

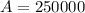
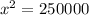
Taking square root on both sides.

`x=500`

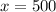
Differentiate with respect to t.

`(dA)/(dt)=2x(dx)/(dt)`

Substitute x=500 and
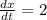`(dx)/(dt)=2` in the above equation.

`(dA)/(dt)=2(500)(2)`

`(dA)/(dt)=2000`

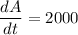
Farmer bob is losing 2,000 square feet of land per year.

Therefore, the correct option is A.