Question

Suppose 42% of politicians are lawyers. If a random sample of size 628 is selected, what is the probability that the proportion of politicians who are lawyers will differ from the total politicians proportion by less than 5%? Round your answer to four decimal places.

Answer 1

0.000024

Explanation:

n = 628

42% of politicians are lawyers

So , Population proportion ,
`\widehat{p}=0.42`

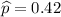
Sample proportion p= 0.5

We are supposed to find
`P(\widehat{p}<0.5)`

Formula :
`z=\frac{p-\widehat{p}}{\frac{\widehat{p}(1-\widehat{p})}{n}}`

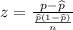
Substitute the values :

`z=\frac{0.5-0.42}{\sqrt{(0.42(1-0.42))/(628)}}`

`z=4.061`

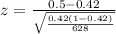
p value = 0.000024

So,the probability that the proportion of politicians who are lawyers will differ from the total politicians proportion by less than 5% is 0.000024