Question

You want to estimate the mean time college students spend watching online videos each day. The estimate must be within 2 minutes of the population mean. Determine the required sample size to construct a 99% confidence interval for the population mean. Assume the population standard deviation is 2.4 minutes.

Answer 1

Answer: 10

Explanation:

We know that the formula to find the sample size is given by :-

`n= ((z_(\alpha/2\cdot \sigma))/(E))^2`

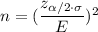
, where
`\sigma` = population standard deviation.

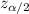`z_(\alpha/2)` = Two -tailed z-value for
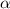`{\alpha` (significance level)

E= margin of error.

Given : Confidence level : C =99%=0.99

i.e.
`1-\alpha=0.99`

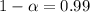
⇒Significance level :
`\alpha=1-0.99=0.01`

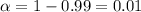
By using z-value table ,Two -tailed z-value for
`\alpha=0.01`:

`z_(\alpha/2)=2.576`

E= 2 minutes

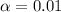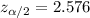
`\sigma=\text{2.4 minutes}`

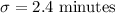
The required sample size will be :-

`n= ((2.576\cdot 2.4)/(2))^2\\\\= (3.0912)^2\\\\=9.55551744\approx10`

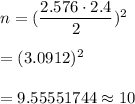
Hence, the required sample size = 10