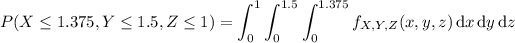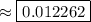Question

Suppose X, Y, and Z are random variables with the joint density function f(x, y, z) = Ce−(0.5x + 0.2y + 0.1z) if x ≥ 0, y ≥ 0, z ≥ 0, and f(x, y, z) = 0 otherwise. (a) Find the value of the constant C. (b) Find P(X ≤ 1.375 , Y ≤ 1.5). (Round answer to five decimal places). (c) Find P(X ≤ 1.375 , Y ≤ 1.5 , Z ≤ 1). (Round answer to six decimal places).

Answer 1

a.

`f_(X,Y,Z)(x,y,z)=\begin{cases}Ce^(-(0.5x+0.2y+0.1z))&\text{for }x\ge0,y\ge0,z\ge0\\0&\text{otherwise}\end{cases}`

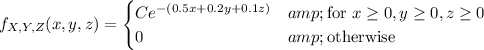
is a proper joint density function if, over its support,
`f` is non-negative and the integral of
`f` is 1. The first condition is easily met as long as
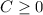`C\ge0`. To meet the second condition, we require

`\displaystyle\int_0^\infty\int_0^\infty\int_0^\infty f_(X,Y,Z)(x,y,z)\,\mathrm dx\,\mathrm dy\,\mathrm dz=100C=1\implies \boxed{C=0.01}`

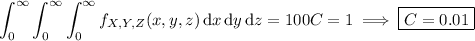
b. Find the marginal joint density of
`X` and
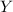`Y` by integrating the joint density with respect to
`z`:

`f_(X,Y)(x,y)=\displaystyle\int_0^\infty f_(X,Y,Z)(x,y,z)\,\mathrm dz=0.01e^(-(0.5x+0.2y))\int_0^\infty e^(-0.1z)\,\mathrm dz`

`\implies f_(X,Y)(x,y)=\begin{cases}0.1e^(-(0.5x+0.2y))&\text{for }x\ge0,y\ge0\\0&\text{otherwise}\end{cases}`

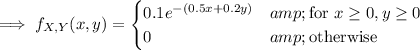
Then

`\displaystyle P(X\le1.375,Y\le1.5)=\int_0^(1.5)\int_0^(1.375)f_(X,Y)(x,y)\,\mathrm dx\,\mathrm dy`

`\approx\boxed{0.12886}`

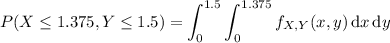
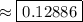
c. This probability can be found by simply integrating the joint density:

`\displaystyle P(X\le1.375,Y\le1.5,Z\le1)=\int_0^1\int_0^(1.5)\int_0^(1.375)f_(X,Y,Z)(x,y,z)\,\mathrm dx\,\mathrm dy\,\mathrm dz`

`\approx\boxed{0.012262}`