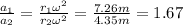Answer:
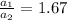
Step-by-step explanation:
The equation for centripetal acceleration is
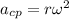 . If
. If
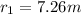 is the distance from the center of the point at the tip of the blade and
is the distance from the center of the point at the tip of the blade and
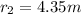 is the distance from the center of another point in the blade, and since both points are rotating at the same angular velocity
is the distance from the center of another point in the blade, and since both points are rotating at the same angular velocity
 because both of them belong to the blade, the ratio between their centripetal accelerations will be:
because both of them belong to the blade, the ratio between their centripetal accelerations will be: