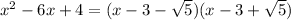Answer:
Part 1)
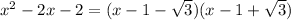
Part 2)
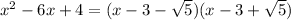
Explanation:
we know that
The formula to solve a quadratic equation of the form

is equal to
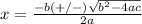
Part 1)
in this problem we have

so
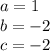
substitute in the formula
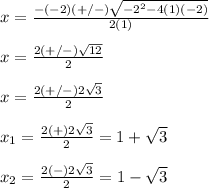
therefore
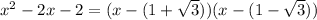
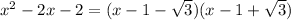
Part 2)
in this problem we have

so
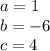
substitute in the formula

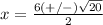
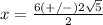
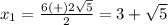
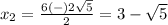
therefore