Question

The distribution of the annual incomes of a group of middle management employees approximated a normal distribution with a mean of $37,200 and a standard deviation of $800. About 68 percent of the incomes lie between what two incomes?

a. $30,000 and $40,000
b. $36,400 and $38,000
c. $34,800 and $39,600
d. $35,600 and $38,800

Answer 1

Answer: b. $36,400 and $38,000

Answer 2

Option B.

Explanation:

Given information:

A group of middle management employees approximated a normal distribution.

Population mean
`\mu` = $37,200

Population standard deviation
`\sigma` = $800

About 68 percent of the incomes lie between two incomes and we need to find those two incomes.

We know that 68% data lies in the interval
`[\mu-\sigma,\mu+\sigma]`.

`\mu-\sigma=37,200-800=36,400`

`\mu+\sigma=37,200+800=38,000`

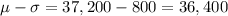
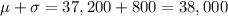
About 68 percent of the incomes lie between what two incomes $36,400 and $38,000.

Therefore, the correct option is B.