Question

The least squares line of best fit for a data set with a positive correlation coefficient always has a:

A. positive slope.
B. positive x-intercept.
C. positive y-intercept.
D. Both A and C are correct.

Answer 1

A. positive slope.

Explanation:

In the least square linear regression of Y on X, the straight line of best fit is given by,

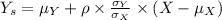`Y_(s) = \mu_(Y) + \rho * \frac {\sigma_(Y)}{\sigma_(X)} * (X - \mu_(X))` ------------------(1)

[where
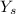`Y_(s)` is the estimated value of Y]

Clearly, here,

Slope pf the line =
`\rho * \frac {\sigma_(Y)}{\sigma_(X)}`---------------------------------(2)

Y- intercept =
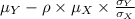`\mu_(Y) - \rho * \mu_(X) * \frac {\sigma_(Y)}{\sigma_(X)}`-----------------(3)

and,

X - intercept =
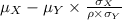`\mu_(X) - \mu_(Y) * \frac {\sigma_(X)}{\rho * \sigma_(Y)}`----------------(4) [putting
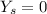`Y_(s) = 0` in (1) and taking the value of X]

So,

since
`\sigma_(Y), \sigma_(X) > 0`

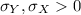
[since
`\sigma_(Y) = 0` or
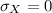`\sigma_(X) = 0` will result in a degenerate distribution, hence these cases are discarded]

so, correlation coefficient =
`\rho` > 0 implies

A. positive slope. [as evident from (1)]

clearly from (3) and (4) all the other options are false.