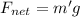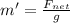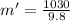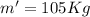Question

A large helium filled balloon is used as the center piece for a graduation party. The balloon alone has a mass of 225 kg and it is filled with helium gas until its volume is 323 m3.

Assume the density of air is 1.20 kg/m3 and the density of helium is 0.179 kg/m3.

(a) Calculate the buoyant force (in N) acting on the balloon. (Enter the magnitude. Round your answer to at least three significant figures.)

(b) Find the net force (in N) on the balloon and determine whether the balloon will rise or fall after it is released. magnitude N direction rise D

(c) What additional mass (in kg) can the balloon support in equilibrium? (If the balloon will fall after it is released, enter 0.)

Answer 1

To solve this problem it is necessary to apply the concepts related to Newton's second law, the definition of density and sum of forces in bodies.

From Newton's second law we understand that

`F= ma (\rightarrow` Gravity at this case)

Where,

m = mass

a= acceleration

Also we know that

`\rho = (m)/(V) \Rightarrow m = \rho V`

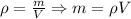
Part A) The buoyant force acting on the balloon is given as

`F_b = ma`

As mass is equal to the density and Volume and acceleration equal to Gravity constant

`F_b = \rho V g`

`F_b = 1.2*323*9.8`

`F_b = 3798.5`

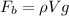
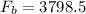
PART B) The forces acting on the balloon would be given by the upper thrust force given by the fluid and its weight, then

`F_(net) = F_b -W`

`F_(net) = F_b -(mg+\rho_H Vg)`

`F_(net) = 3798.5-(9.8*225*9.8*0.179*323)`

`F_(net) = 1030N`

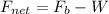
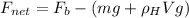
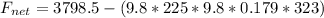
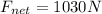
PART C) The additional mass that can the balloon support in equilibrium is given as

`F_(net) = m' g`

`m' =(F_(net))/(g)`

`m' = (1030)/(9.8)`

`m' = 105Kg`