Question

You hear a sound with a frequency of 256 Hz. The amplitude of the sound increases and decreases periodically: it takes 2 seconds for the sound to go from loud to soft and back to loud. This sound can be thought of as a sum of two waves with frequencies:

(A) 256 Hz and 2 Hz.
(B) 254 Hz and 258 Hz.
(C) 255 Hz and 257 Hz.
(D) 255.5 Hz and 256.5 Hz.
(E) 255.75 Hz and 256.25 Hz.

Answer 1

To solve this problem it is necessary to take into account the concepts related to frequency and period, and how they are related to each other.

The relationship that defines both agreements is given by the equation,

`f_(beat)=(1)/(T)`

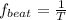
Then the frequency for the previous period given (2sec) is

`f_(beat)=(1)/(2)`

`f_(beat) = 0.5Hz`

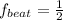
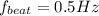
The beat frequency of two frequencies is equal to the difference between the two frequencies, then

`f_(beat) = |f_1-f_2|\\f_(beat) = |256Hz-2Hz|\\f_(beat) = 254Hz`

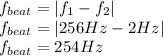
Hence option A is incorrect.

We can do this process for 254Hz as
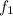`f_1` and 258 Hz for
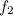`f_2` , then

`f_(beat) =|254Hz-258Hz|`

`f_(beat) = 4Hz`

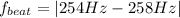
Hence option B is incorrect.

We can also do this process for 255Hz as
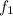`f_1` and 257 Hz for
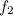`f_2` , then

`f_(beat) =|255Hz-257Hz|`

`f_(beat) = 2Hz`

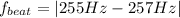
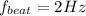
Hence option C is incorrect.

We can also do this process for 255.5Hz as f_1 and 256.5 Hz for f_2, then

`f_(beat) =|255.5Hz-256.5Hz|\\f_(beat) = 1Hz`

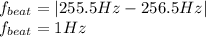
Hence option D is incorrect.

We can also do this process for 255.75Hz as
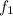`f_1` and 256.25 Hz for
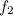`f_2`, then

`f_(beat) =|255.75Hz-256.25Hz|\\f_(beat) = 0.5Hz`

Hence option E is incorrect.

Therefore the sum of the frequencies in the sound wave would be 256.25Hz and 255.75Hz