Question

The function f(x) has been transformed to give g(x). Which of the following functions represent g(x)

A. g(x) = (1/2) x^2
B. g(x) = 4x^2
C. g(x) = 1/4 x^2
D. g(x) = 2x^2
f(x) = x2
g(x) = ?

Answer 1

The answer is g(x) = (1/2) x^2

Explanation:

Answer 2

Option A is correct.

Explanation:

See the two graphs given in the diagram attached.

The first graph shows the function f(x) = x²

Hence, it passes through the points (1,1), (-1,1), (2,4) and (-2,4) points.

Now, from the plotted second graph we see the points (2,2), and (-2,2) are on the curve.

Hence, the y-value corresponding to the same x-value reduces by a factor
`(1)/(2)` in the second graph i.e. the graph of y = g(x) compared to the first graph.

So, we can conclude that the transformed graph has the equation
`g(x) = (1)/(2) x^(2)`.

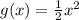Hence, option A is correct. (Answer)