Question

Which equation has infinitely many solutions?

A. 12 + 4x = 6x + 10 – 2x
B. 5x + 14 – 4x = 23 + x – 9
C. x + 9 – 0.8x = 5.2x + 17 – 8
D. 4x – 2x = 20

Answer 1

An equation has infinitely many solutions if it can be manipulated all the way to an identity (i.e. an equality where the right and left hand side are the same). We have:

A)
`12+4x=6x+10-2x \iff 12+4x=10+4x \iff 12=10`

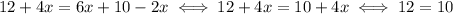
which is impossible

B)
`5x+14-4x=23+x-9 \iff x+14 = x+14`

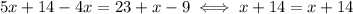
which is an equality

C)
`x+9-0.8x=5.2x+17-8\iff 0.2x+9=5.2x+9 \iff 0.2x=5.2x\iff x=0`

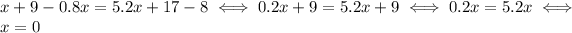
which has a unique solution

D)
`4x-2x=20 \iff 2x=20 \iff x=10`

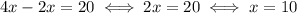
which has a unique solution